Twinning
Definition
"Twins are regular aggregates consisting of crystals of the same species joined together in some definite mutual orientation" (Giacovazzo, 2002). So for the description of a twin two things are necessary: a description of the orientation of the different species relative to each other (the twin law) and the fractional contribution of each component. The twin law can be expressed as a matrix that transforms the hkl indices of one species into the other.
Classification
Depending on the twin law four types of twins can be distinguished:
Twinning by Merohedry
In a merohedral twin, the twin law is a symmetry operator of the crystal system, but not of the point group of the crystal. This means that the reciprocal lattices of the different twin domains superimpose exactly and the twinning is not directly detectable from the reflection pattern. This type is possible in the trigonal, tetragonal, hexagonal and cubic crystal systems, which have more than one Laue group. The twin law corresponds to the two-fold operation that is present in the apparent Laue group, but not in the true space group.
Twinning in P3, P4, P6 and cubic crystals often involves only two domains (hemihedry). Only for trigonal crystals is there more than one possible twin law. Higher forms of merohedral twinning then exist: tetartohedry (4 twin domains) and ogdohedry (8 twin domains).
Twinning by Pseudo-Merohedry
In a pseudo-merohedral twin, the twin operator belongs to a higher crystal system than the structure. This may happen if the metric symmetry is higher than the symmetry of the structure. Depending on how well the higher metric symmetry is fulfilled, it may happen that the reciprocal lattices overlap exactly and the twinning is not detectable from the diffraction pattern. But, compared to merohedral twins,the number of possible twin laws is much higher.
Twinning by Reticular Merohedry
Some of the reflections overlaps exactly, while others are non-overlapped. A typical example is an obverse/reverse twin in case of a rhombohedral crystal. Thus, this kind of twinning lets R3 look like P31,2, and R32 like P31,221. However, 1/3 of all reflections are still zero.
Non-Merohedral Twins
For non-merohedral twins, the twin law does not belong to the crystal class of the structure nor to the metric symmetry of the cell. Therefore the different reciprocal lattices do not overlap exactly. There are three types of reflections, non-overlapped, partially overlapped and exactly overlapped reflections. Here the problems start in the data collection. If both twin domains are similar in size, there are often problems with the cell determination and usual automatic indexing programs fail. More than one orientation matrix is needed to index all reflections. In the integration process the information of all matrices should be used.
Solution of twinned structures by experimental phasing
Yates & Rees, Acta Cryst. (1987). A43, 30-36 give a method for solving a twinned structure using MIR. This requires four independent derivatives.
Refinement
Refinement of merohedrally and pseudo-merohedrally twinned data data is possible using CNS (for input files, see CNS homepage - main menu - input files - x ray - twinning), Refmac or phenix.refine (Refinement using twinned data) and has "always" been possible with SHELXL (Twin-Refinement with SHELXL).
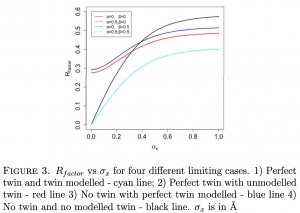
Be aware that R-factors may not be directly comparable: G. Murshudov, Appl. Comput. Math., V.10, N.2, 2011, pp.250-261 (see figure)
Warning Signs for Twinning
Experience shows that there are a number of characteristic warning signs of twinning, as given in the following list. Of course not all of them can be present in any particular example, but if one or several apply, the possibility of twinning should be given serious consideration.
- The metric symmetry is higher than the Laue symmetry.
- The Rint-value for the higher symmetry Laue group is only slightly higher than for the lower symmetry Laue group.
- If different crystals of the same compound show significantly different Rint values for the higher symmetry Laue group, this clearly shows that the lower symmetry Laue group is correct and indicates different extents of twinning.
- The mean value for |E^2-1| is much lower than the expected value of 0.736 for the non-centrosymmetric case (see also Intensity statistics). If we have two twin domains and every reflection has contributions from both, it is unlikely that both contributions will have very high or that both will have very low intensities, so the combined intensities are distributed to give fewer extreme values.
- The space group appears to be trigonal or hexagonal.
- The apparent systematic absences are not consistent with any known space group.
- Although the data appear to be in order, the structure cannot be solved. This may of course also happen if the cell is wrong, for example with an halved axis
- The cell volume is too small for the size of the molecule, or the Patterson function is physically impossible.
The following features are typical of non-merohedral twins, where the reciprocal lattices do not overlap exactly and only some of the reflections are affected by the twinning:
- There appear to be one or more unusually long axes.
- There are problems with the unit cell refinement.
- Some reflections are sharp, others split.
- K = mean(Fo^2)/mean(Fc^2) is systematically high for reflections with low intensity. This may also indicate a wrong choice of space group in the absence of twinning.
References
Giacovazzo, C. ed. (2002). Fundamentals in Crystallography, I.U.Cr. & O.U.P.: Oxford, UK.
Other Helpful Papers on Twinning
Parsons, S. Introduction to twinning. Acta Cryst (2003) D59, 1995-2003.
Dauter, Z. Twinned crystals and anomalous phasing. Acta Cryst (2003) D59, 2004-2016
Examples, with background references
Detecting and overcoming hemihedral twinning during the MIR structure determination of Rna1p. Hillig RC, Renault L. Acta Crystallogr D Biol Crystallogr. 2006 Jul;62(Pt7):750-65.
Other Crystal Pathologies
Zwart, PH et al. Surprises and pitfalls arising from (pseudo)symmetry. Acta Cryst (2008) D64, 99-107.